Vaida's treatment of the verbal morphology involves a tiny handful of intriguing isomorphisms, surrounded by an impenetrable sea of assumptions and highly controversial internal reconstructions that create an illusion of systemic reconstruction where there really is none.

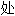
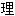
Vaida 对词语形态学的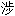及极少数有趣的同构，而这些同构周围则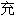满了难以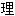解的假设和极具争议的内部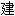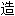， 成了一种系统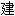的假象， 但实际上并不存在这种。
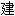